Introduction
Hello there, I hope you’re doing well. You’ve already read about the knapsack problem & its solution using the Greedy method. In this blog, you’ll learn to solve the 0/1 knapsack problem using dynamic programming. So, keep reading till the end of the blog for the solution.
Let’s get started…..
Knapsack problem
Given a knapsack of maximum capacity M kg and N items with its own weight and profit. Fill in the knapsack with a subset of items such that the weight is less than or equal to the limit of the knapsack and the value of items is maximum.
0/1 Knapsack Problem
In this either the whole item can be selected(1) or not selected at all(0) i.e. we can select a portion of any item according to the need. We can choose not some portion of any item as and when needed.
Max z=∑ni=1pi*xi
S.t. ∑ni=1wi≤m
And xi=1 or 0
Where, p=profit, w=weight, x=solution or selection value,m=capacity and s.t.=subject to
0/1 Knapsack problem using Dynamic Programming:
In this problem, we have a Knapsack that has a capacity m.
There are items i1,i2,….,in each having weight w1,w2,…..wn and some benefit(value or profit) associated with it p1,p2,….,pn
Our objective is to maximize the benefit such that the total weight inside the Knapsack is at most m.
Since this is a 0-1 Knapsack problem, we can either take an entire item or reject it completely. We cannot break an item and fill the Knapsack.
To solve 0/1 knapsack problem using dynamic programming use the following recursive formula:-
fn(m)=max[fn-1(m);fn-1(m-wn)+pn]
fn(-m)=-∞
f0(m)=0
Example:-
Assume that we have a Knapsack with max weight capacity m=8.
Our objective is to fill the Knapsack with items such that the benefit (profit) is maximum.
| Item i | 1 | 2 | 3 |
| Profit p | 15 | 20 | 21 |
| Weight w | 5 | 4 | 3 |
Total items i.e. n=3
First apply this formula:- fn(m)=max[fn-1(m);fn-1(m-wn)+pn]
Set-1:- f3(8)=max[f2(8);f2(8-3)+21] …………………….1
f2(8)=max[f1(8);f1(4)+20] ………………………2
f2(5)=max[f1(5);f1(1)+20] ……………………….3
f1(8)=max[f0(8);f0(3)+15] ………………………..4
f1(4)=max[f0(4);f0(-1)+15] ……………………….5
f1(5)=max[f0(5);f0(0)+15] ………………………..6
f1(1)=max[f0(1);f0(-4)+15] ………………………..7
Apply:- fn(-m)=-∞
and f0(m)=0
Set-2:- f0(8)=0……………………………………8
f0(3)=0…………………………………….9
f0(4)=0……………………………………10
f0(-1)=-∞…………………………………11
f0(5)=0……………………………………12
f0(0)=0……………………………………13
f0(1)=0……………………………………14
f0(-4)=-∞…………………………………15
Set-3:- Put equation 8 to 15 into 4 to 7:-
f1(8)=max[0;0+15]=15………………………….16
f1(4)=max[0;-∞+15]=0………………………….17
f1(5)=max[0;0+15]=15………………………….18
f1(1)=max[0;-∞+15]=0………………………….19
Set-4:- Put equation 16 to 19 into 2 and 3 :-
f2(8)=max[15;0+20]=20………………………….20
f2(5)=max[15;0+20]=20………………………….21
Set-5:- Put equation 20 and 21 into 1:-
F3(8)=max[20;20+21]
max[20,41]
Maximum profit=41
Program to solve 0/1 Knapsack problem using dynamic programming:
#include<stdio.h>
#include<conio.h>
int max(int a,int b){return(a>b)?a:b;}
int KnapSack(int W,int wt[],int val[],int n)
{
int i,w;
int K[20][20];
for(i=0;i<=n;i++)
{
for(w=0;w<=W;w++)
{
if(i==0||w==0)
K[i][w]=0;
else if(wt[i-1]<=w)
K[i][w]=max(val[i-1]+K[i-1][w-wt[i-1]],K[i-1][w]);
else
K[i][w]=K[i-1][w];
}
}
return K[n][W];
}
void main()
{
int i,n,val[20],wt[20],W;
clrscr();
printf(“Enter number of items:”);
scanf(“%d”,&n);
printf(“Enter value and weight of items:\n”);
for(i=0;i<n;++i)
{ scanf(“%d%d”,&val[i],&wt[i]);
}
printf(“Enter size of Knapsack:”);
scanf(“%d”,&W);
printf(“%d”,KnapSack(W,wt,val,n));
getch();
}
Output:-
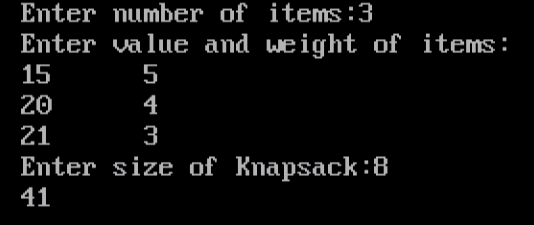
Blog By:- Mr. Rahul Agarwal (Assistant Professor)
Department Of Information Technology
Biyani Group Of Colleges, Jaipur
CLICK HERE to pursue your Graduation by sitting at your home.

